Extend the power rule to functions with negative exponents. Example of polynomial function.

Polynomials Intro Video Khan Academy
We commonly associate the Power Rule with polynomials but it works for any expression in the form xn.
. Roots are at x2 and x4. Polynomials in one variable are algebraic expressions that consist of terms in the form axn a x n where n n is a non-negative ie. Polynomials are also an essential tool in describing and predicting traffic patterns so appropriate traffic control measures such as traffic lights can be implemented.
7a2b 3b2 a2b 2. Polynomials are terminating expressions that can be expressed by constants and indeterminates using the binary operations of addition subtraction and multiplication. Q2If sum of the zeroes of the polynomial x2-x-k2x-1 is 0find the value of k Q3If 2 and 3 are the zeroes of the polynomial 3x2-2kx2m find the values of k.
However when one considers the function defined by the polynomial then x represents the argument of the function and is therefore called a variable. In this process the surfaces that are rendered are made from solutions to algebraic. A simple feedback rule will be more robust to rescaling.
Recently researchers have been investigating the use of polynomials for rendering graphics in part because it demands less memory in the process. Use special product rules to multiply a binomial squared and a product of a sum and difference of two terms. Want a deeper explanation.
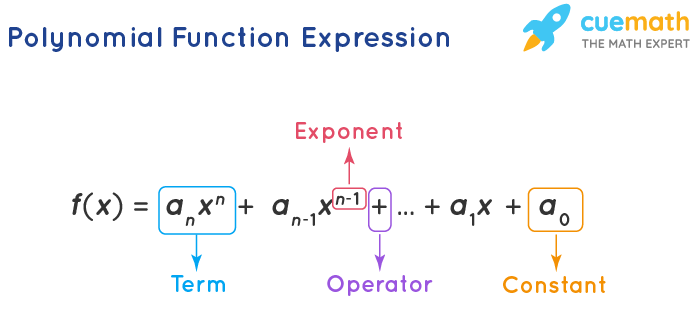
Describe the end behavior of f x 3x7 5x 1004. A plain number can also be a polynomial term. Fx 3x 2 5x 19.
Ad Over 27000 video lessons and other resources youre guaranteed to find what you need. Sometimes its useful to have a way to express a variable as a flexible function of time so that you can find the trajectory that maximizes some quantity like profit or fit to data. Continue reading Polynomials Interpolating Functions for Decision Rules.
Usually the polynomial equation is expressed in the form of a n x n. Polynomials cannot contain division by a variable. 21 is a polynomial.
Q1If one zero of the polynomial 3x2-kx-2 is 2 find the other zeroallso find the value of k. It has just one term which is a constant. 5 13 21 29 37 45 18n800 28n-3797 33n-8292 458n805 I think 4 is correct.
The Rule of Signs. Polynomial identities can be used to explain or describe various things in math. This is not generally the best thing to do.
Use the FOIL method to multiply a binomial times a binomial. Then find the 100th term in the sequence. In this tutorial we will be looking at the different components of polynomials.
The graph of a polynomial function can also be drawn using turning points intercepts end behaviour and the Intermediate Value Theorem. To differentiate polynomials all you need are the Power rule and the basic differentiation rules. Combine the differentiation rules to find the derivative of a polynomial or rational function.
There are a few rules as to what polynomials cannot contain. Example of a polynomial. Lets differentiate for example.
This is the currently selected item. These are the most important rules for multiplication of polynomials. To multiply a number by a polynomial we use the distributive property.
Here a is the. For example polynomials can be used to figure how much of a gardens surface area can be covered with a certain amount of soil. We then add the products together.
Below are images of smooth-surfaced objects that were rendered using polynomials by researchers. This polynomial is much too large for me to view in the standard screen on my graphing calculator so either I can waste a lot of time fiddling with WINDOW options or I can quickly use my knowledge of end behavior. Recently researchers have been investigating the use of polynomials for rendering graphics in part because it demands less memory in the process.
Polynomials cannot contain negative exponents. Technically terms can only be called polynomial terms if the power n is a non-negative integer. For example we can use identities to prove that the differences between consecutive square numbers 149 are consecutive odd numbers 135.
A special way of telling how many positive and negative roots a polynomial has. For example 2y27x4 is a polynomial because 4 is not a variable. Up to 24 cash back polynomial equation can be used in any 2-D construction situation to plan for the amount of materials needed.
However the Power Rule works for any expression xn where n is. Xy4 5x2z has two terms and three variables x y and z. This definition reflects the historical approach to polynomials since they far predate the formal notion of.
In this process the surfaces that are rendered are made from solutions to algebraic polynomials. Polynomial are sums and differences of polynomial terms. Polynomials can have no variable at all.
Briefly describe what seems to be occurring as the number of hours increases. Economists use polynomials to model economic growth patterns and medical researchers use them to describe the behavior of bacterial colonies. The degree of a polynomial in one variable is the largest exponent in the polynomial.
Polynomials have roots zeros where they are equal to 0. The same method applies to many flat-surface projects including driveway sidewalk and patio construction. Write an expression to describe a rule for the sequence.
If it is a polynomial find the degree and determine whether it is a monomial binomial or trinomial. This one has 3 terms. This method of rendering graphics works well and is in wide use but it takes a lot of computer memory.
You cannot have 2y-27x-4. This function is an odd-degree polynomial so the ends go off in opposite. Positive or zero integer and a a is a real number and is called the coefficient of the term.
For an expression to be a polynomial term any variables in the expression must have whole-number powers or else the understood power of 1 as in x1 which is normally written as x. Answer 1 of 4. Multiplying polynomials is a bit more complicated because you have more than two factors which contain more than one term.
Multiply any polynomial times any other polynomial. X4 2x2 x has three terms but only one variable x Or two or more variables. We can distribute the in to obtain the equivalent expression When multiplying polynomials the distributive property allows us to multiply each term of the first polynomial by each term of the second.
The x occurring in a polynomial is commonly called a variable or an indeterminateWhen the polynomial is considered as an expression x is a fixed symbol which does not have any value its value is indeterminate. The number must be distributed to each term of the polynomial. However 2y27x 1x is not a polynomial as it contains division by a variable.
Use the quotient rule for finding the derivative of a quotient of functions. A Polynomial looks like this.

Polynomial Function Definition Formulas Types Graph
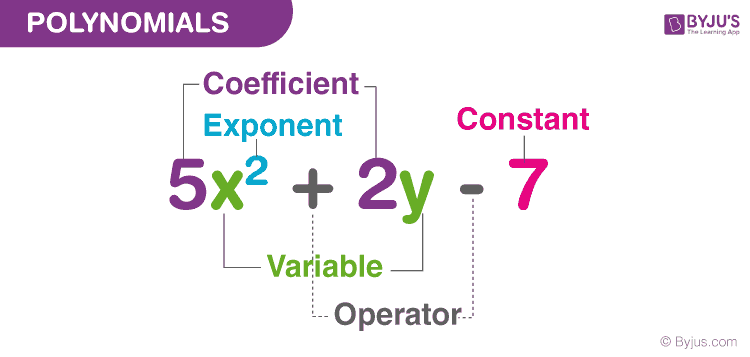
Polynomials Definition Types And Examples
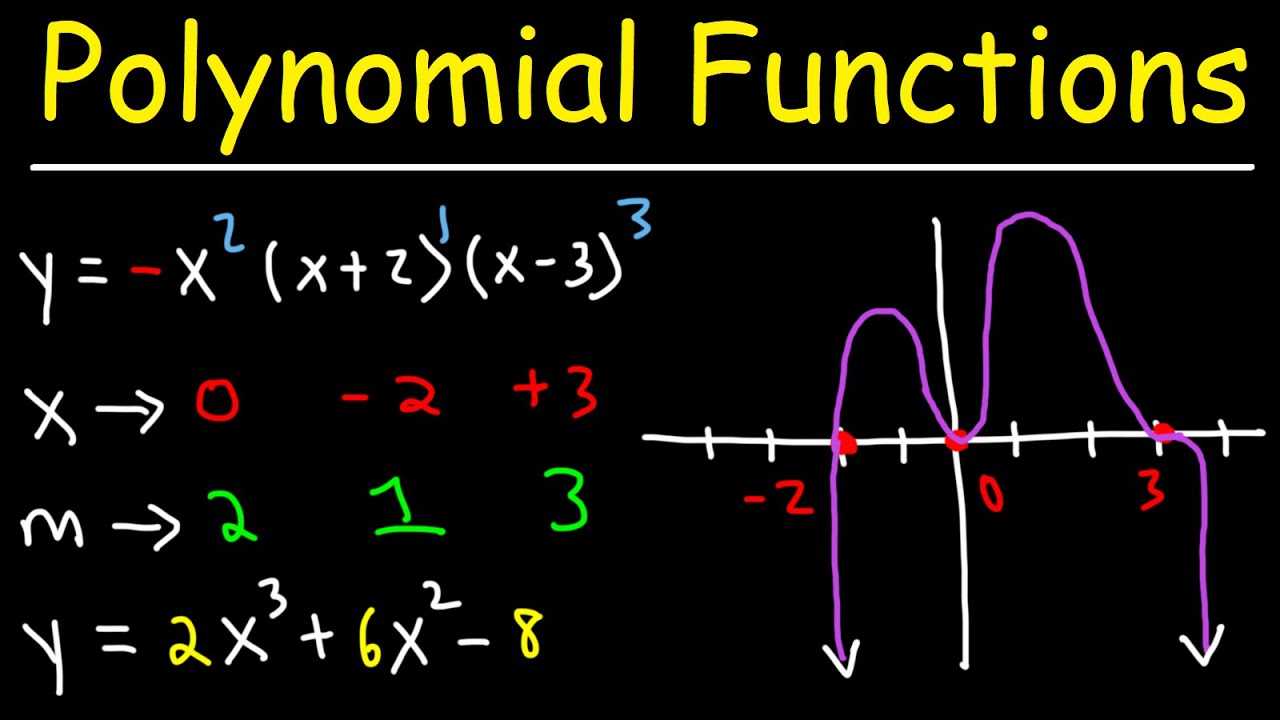
How To Graph Polynomial Functions Using End Behavior Multiplicity Zeros Youtube
0 Comments